Next: Imaging Up: Single-field imaging and deconvolution Previous: Single-field imaging and deconvolution Contents Index

Measurement equation and other definitions

The measurement equation of an instrument is the relationship between the sky intensity and the measured quantities. The measurement equation for a millimeter interferometer is to a good approximation (after calibration)

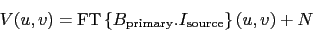$\begin{displaymath} V(u,v) = \mbox{FT}\ensuremath{\displaystyle\left\{ B_\ensur... ...thrm{primary}}.I_\ensuremath{\mathrm{source}} \right\}}(u,v)+N \end{displaymath}$

(4.1)

where $\mbox{FT}\ensuremath{\displaystyle\left\{ F \right\}}(u,v)$ is the bi-dimensional Fourier transform of the function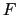

taken at the spatial frequency

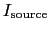, $I_\ensuremath{\mathrm{source}}$ the sky intensity distribution, $B_\ensuremath{\mathrm{primary}}$ the primary beam of the interferometer (i.e. a Gaussian whose FWHM is the natural resolution of the single-dish antenna composing the interferometer),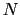

some thermal noise and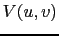

the calibrated visibility at the spatial frequency

. This measurement equation implies different kinds of problems.

The presence of noise leads to sensitivity problems.
The multiplication of the sky intensity by the primary beam implies a distortion of the information about the intensity distribution of the source.
The presence of the Fourier transform implies that visibilities belongs to the Fourier space while most (radio)astronomers are used to work in the image space. A step of imaging is thus required to go from the 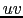plane to the image plane.
Finally, the main problem implied by this measurement equation is certainly the irregular, limited sampling of the 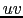plane because it implies that the information about the source intensity distribution is incomplete.

To show that deconvolution techniques are needed to overcome the incomplete sampling of the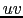

plane, we need additional definitions

Let's call $V = \mbox{FT}\ensuremath{\displaystyle\left\{ B_\ensuremath{\mathrm{primary}}.I_\ensuremath{\mathrm{source}} \right\}}$ the continuous visibility function.
The sampling function 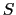is defined as
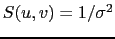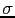- $S(u,v) = 1/\sigma^2$ at spatial frequencies where visibilities are measured by the interferometer. $\sigma{}$ is the rms noise predicted from the system temperature, antenna efficiency, integration time and bandwidth. The sampling function thus contains information on the relative weights of each visibility.
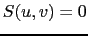- elsewhere.
We finally call 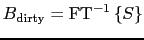$B_\ensuremath{\mathrm{dirty}} = \mbox{FT}^{-1} \ensuremath{\displaystyle\left\{ S \right\}}$ the dirty beam.

If we forget about the noise, we can thus rewrite the measurement equation as

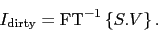$\begin{displaymath} I_\ensuremath{\mathrm{dirty}} = \mbox{FT}^{-1} \ensuremath{\displaystyle\left\{ S.V \right\}}. \end{displaymath}$

(4.2)

Using the property #1 of the Fourier transform (see Appendix), we obtain

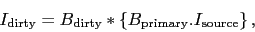$\begin{displaymath} I_\ensuremath{\mathrm{dirty}} = B_\ensuremath{\mathrm{dirty... ...th{\mathrm{primary}}.I_\ensuremath{\mathrm{source}} \right\}}, \end{displaymath}$

(4.3)

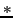where $\ast$ is the convolution symbol. Thus, the incompleteness of the

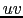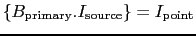sampling translate in the image plane as a convolution by the dirty beam, implying the need of deconvolution. From the last equation, it is easy to show that the dirty beam is point spread function of the interferometer, i.e. its response at a point source. Indeed, for a point source at the phase center, $\ensuremath{\displaystyle\left\{ B_\ensuremath{\mathrm{primary}}.I_\ensuremath{\mathrm{source}} \right\}} = I_\ensuremath{\mathrm{point}}$ at the phase center and 0 elsewhere and the convolution with a point source is equal to the simple product: 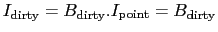$I_\ensuremath{\mathrm{dirty}} = B_\ensuremath{\mathrm{dirty}}.I_\ensuremath{\mathrm{point}} = B_\ensuremath{\mathrm{dirty}}$ for a point source of intensity 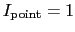$I_\ensuremath{\mathrm{point}} = 1$ Jy.

Next: Imaging Up: Single-field imaging and deconvolution Previous: Single-field imaging and deconvolution Contents Index

Gildas manager 2014-07-01