




Next: Mapping
Up: Sensitivity
Previous: Sensitivity
Contents
Index
The point source sensitivity (one 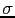 level)
for an
level)
for an 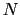 antenna array, over a bandwidth
antenna array, over a bandwidth 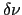 in a time
in a time
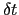 is given by
is given by
 |
(1) |
where
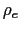 is the antenna efficiency
(Jy K
is the antenna efficiency
(Jy K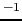 ) i.e.
) i.e.
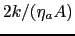 with
with 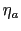 the aperture
efficiency,
the aperture
efficiency, 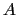 the antenna collecting area, and
the antenna collecting area, and 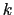 the Boltzmann
constant (22Jy.K
the Boltzmann
constant (22Jy.K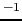 at 85GHz, 29Jy.K
at 85GHz, 29Jy.K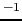 at 150GHz,
35Jy.K
at 150GHz,
35Jy.K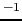 at 230GHz and 45 Jy/K at 350GHz),
at 230GHz and 45 Jy/K at 350GHz),
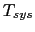 is the system temperature
outside the atmosphere, i.e. corrected for atmospheric transmission
(typically 100K SSB below 110 GHz, but 180K (170K) at 115 GHz,
150K (130K) below 150GHz, 200K (170K) above 150GHz,
and 250K (200K) at 230 GHz in summer (values in brackets are for
typical winter conditions)). Use
is the system temperature
outside the atmosphere, i.e. corrected for atmospheric transmission
(typically 100K SSB below 110 GHz, but 180K (170K) at 115 GHz,
150K (130K) below 150GHz, 200K (170K) above 150GHz,
and 250K (200K) at 230 GHz in summer (values in brackets are for
typical winter conditions)). Use 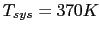 at 350GHz,
at 350GHz,
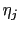 is the instrumental decorrelation coefficient, related to
the oscillator phase jitter by
is the instrumental decorrelation coefficient, related to
the oscillator phase jitter by
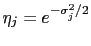 (0.90 to
0.98),
(0.90 to
0.98),
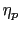 is the atmospheric decorrelation coefficient, related to
the atmospheric phase noise by
is the atmospheric decorrelation coefficient, related to
the atmospheric phase noise by
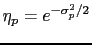 (0.6 to
0.98),
(0.6 to
0.98),
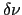 is the noise equivalent bandwidth of the correlator in Hz,
equal to 1.88 times the channel separation; the effective channel
width for standard apodization (using a Welch time-lag window) is
1.60 times the channel separation,
is the noise equivalent bandwidth of the correlator in Hz,
equal to 1.88 times the channel separation; the effective channel
width for standard apodization (using a Welch time-lag window) is
1.60 times the channel separation,
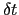 is the on-source integration time per configuration
in seconds (2 to 8 hours, depending on source declination). Because of
various calibration observations, the total observing time is typically
1.6
is the on-source integration time per configuration
in seconds (2 to 8 hours, depending on source declination). Because of
various calibration observations, the total observing time is typically
1.6 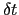 , and
, and
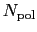 is the number of polarizations: 1 for single
polarization and 2 for dual polarization (see Section
is the number of polarizations: 1 for single
polarization and 2 for dual polarization (see Section ![[*]](crossref.png) for
details).
for
details).
The instrumental phase jitter 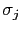 typically ranges between 3
and 5 degrees at 90 GHz, and increases linearly with frequency. The
short and long term atmospheric phase fluctuations
typically ranges between 3
and 5 degrees at 90 GHz, and increases linearly with frequency. The
short and long term atmospheric phase fluctuations  depend on the baseline length and water vapor content of the atmosphere.
15 degrees is a typical value for short (
depend on the baseline length and water vapor content of the atmosphere.
15 degrees is a typical value for short (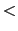 100m) baselines.
100m) baselines.
With typical values for Plateau de Bure and assuming 6 antennas, we
find at 90 GHz 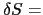 0.08mJy (
0.08mJy (
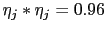 ) to
0.15mJy (
) to
0.15mJy (
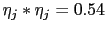 ) for a one hour integration in
a 3600MHz bandwidth and using both polarizations. For a full
synthesis (2 configurations, or 11 hours on source), this goes down to
about 0.03mJy (
) for a one hour integration in
a 3600MHz bandwidth and using both polarizations. For a full
synthesis (2 configurations, or 11 hours on source), this goes down to
about 0.03mJy (
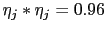 ) to 0.04mJy (
) to 0.04mJy (
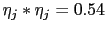 ). At 115 GHz, the sensitivity is degraded by
about a factor 2 because of higher system noise and atmospheric phase
fluctuations. At 230 GHz, the expected rms noise is 0.26 to 0.53mJy in
one hour (0.08 mJy to 0.14 mJy after 11 hours in average winter
conditions).
). At 115 GHz, the sensitivity is degraded by
about a factor 2 because of higher system noise and atmospheric phase
fluctuations. At 230 GHz, the expected rms noise is 0.26 to 0.53mJy in
one hour (0.08 mJy to 0.14 mJy after 11 hours in average winter
conditions).





Next: Mapping
Up: Sensitivity
Previous: Sensitivity
Contents
Index
Gildas manager
2014-07-01
![]() level)
for an
level)
for an ![]() antenna array, over a bandwidth
antenna array, over a bandwidth ![]() in a time
in a time
![]() is given by
is given by

![[*]](crossref.png) for
details).
for
details).
![]() typically ranges between 3
and 5 degrees at 90 GHz, and increases linearly with frequency. The
short and long term atmospheric phase fluctuations
typically ranges between 3
and 5 degrees at 90 GHz, and increases linearly with frequency. The
short and long term atmospheric phase fluctuations ![]() depend on the baseline length and water vapor content of the atmosphere.
15 degrees is a typical value for short (
depend on the baseline length and water vapor content of the atmosphere.
15 degrees is a typical value for short (![]() 100m) baselines.
100m) baselines.
![]() 0.08mJy (
0.08mJy (
![]() ) to
0.15mJy (
) to
0.15mJy (
![]() ) for a one hour integration in
a 3600MHz bandwidth and using both polarizations. For a full
synthesis (2 configurations, or 11 hours on source), this goes down to
about 0.03mJy (
) for a one hour integration in
a 3600MHz bandwidth and using both polarizations. For a full
synthesis (2 configurations, or 11 hours on source), this goes down to
about 0.03mJy (
![]() ) to 0.04mJy (
) to 0.04mJy (
![]() ). At 115 GHz, the sensitivity is degraded by
about a factor 2 because of higher system noise and atmospheric phase
fluctuations. At 230 GHz, the expected rms noise is 0.26 to 0.53mJy in
one hour (0.08 mJy to 0.14 mJy after 11 hours in average winter
conditions).
). At 115 GHz, the sensitivity is degraded by
about a factor 2 because of higher system noise and atmospheric phase
fluctuations. At 230 GHz, the expected rms noise is 0.26 to 0.53mJy in
one hour (0.08 mJy to 0.14 mJy after 11 hours in average winter
conditions).